
Fig 1. Dune migration by avalanching (press Esc to stop animation, PageRefresh to resume)
Dunes can migrate by avalanching (figure 1). Wind scours sand from the stoss slope and blows it over the crest, where it accumulates in a cornice that eventually becomes too steep and avalanches.

Fig 1. Dune migration by avalanching (press Esc to stop animation, PageRefresh to resume)
If not all the sand is scoured away behind an advancing dune, a set (ie., layer) of cross-strata can be left (figure 1). If subsequent dune troughs also do not scour away that set, it becomes preserved, appearing stratigraphically as cross-strata between two bounding surfaces.
Seasonal wind reversals can leave annual depositional wedges (figure 2):

Fig 2. Seasonal cycles of deposition can leave annual depositional wedges.
Figure 3, below, is a photo of cross-stratified sets of Jurassic Navajo Sandstone at Coyote Buttes in south Utah, USA. The thick cross-strata are interpreted as being annual depositional wedges, created by seasonal wind reversals. Counting the number of annual depositional wedges in a set, it took about 50 years for a dune to migrate across the distance in the photo.

Fig 3. Outcrop with about a dozen sets of crossbedding (Coyote Buttes, Utah) (flipped left-to-right to match fig 1 and 2)
There are about a dozen sets, each about two or three meters thick. So how long did it take to deposit that stack of sets? Did every migrating dune leave a set? How many dunes passed without leaving a set, or eroding earlier sets? What percentage of the dunes that passed left deposits that contributed to this stratigraphic record?
Given characteristics of the sets, what can be deduced about the dunes that deposited these sets?

Fig 4. Screenshot of dune stratigraphy model
This computer model explores the stratigraphy created by a simulated train of migrating dunes. The focus is on the roughly-horizontal bounding surfaces that delimit the diagonal cross-strata features; the cross-strata themselves, deposited by slipface avalanches, are not modelled. Thus the dune is an instance of a more generalized bedform, with focus upon the time-space history of the trough between bedforms.
Each bedform in the model is defined by the location of its peak and trough, and these are connected with simple sinusoidal curves. Bedforms are migrated left to right. Bedforms are created at the left edge, and assigned a height. Aggradation is accomplished by incrementing the height of newly-created peaks. Trough depth is initialized relative to the peak, and can slowly vary, sinusoidally, as the bedform migrates rightward. Trough trajectories define the stratigraphy and are made visible by tracing the path of troughs (black lines).
Whatever process controls the depth of scour by wind gusts as an aeolian dune migrates, it probably has an essentially-stochastic component. The purpose here is to get a feel for the effects of random jitter in parameters of a simplified model of 'bedform migration' upon the resulting 'stratigraphic record', drawing upon fluvial processes research.
The model's output has some resemblance to natural crossbed bounding surfaces and to the output of more sophisticated computer models, eg., as described by Leclair (2002) where topology and trough movements are based on observations of subaqueous dunes in laboratory flumes, and by Jerolmack (2005) where topology and trough movements are based on phenomenological continuum models of the dynamics of granular flow.
This model assumed a homogeneous, erodible substrate. Of course in nature, trough depth is probably frequently constrained by inhomogeneities of the environment, eg., a hard interdune surface, a water table, or other variations in cohesion.
Various model scenarios and resulting stratigraphy are discussed in the sections below.
Figure 5 illustrates a series of aggradating bedforms leaving parallel climbing translatent strata (sets) as they migrate to the right. Trailing black lines mark the earlier positions of each trough. In figure 5 below, the black lines begin at what were the initial positions of the bedforms when the model was initialized, and here the model was run for time only long enough for migration of about five bedform wavelengths.
Each bedform's trough marks the erosion of all but a small amount of the preceding bedform's deposit; each dune appears to 'climb' upon the remaining sand (which becomes a thin strata) of the preceding dune. The net effect is aggradation, a rise in the mean level of the bedform surface.
This scenario is often found in early or simplified literature (and figures 1 and 2, above) discussing cross-strata sets (usually shown climbing). Its non-stochastic regularity also underlies many cyclic stratigraphy models.

Fig 5. Climbing translatent strata, produced by bedforms of fixed geometry and equal troughs
Dunes migrate by sand eroding from their stoss side (left, here) and depositing on their lee face (right, here). This can persist indefinitely if the stoss-side erosion balances the leeside-deposition. But if there is net deposition due to incomplete erosion on the stoss side (as in figure 5), to persist indefinitely each bedform in the train would need to acquire new sand compensating for what it left behind (or more, in which case it would grow). Bedforms in a train could possibly obtain new sand (to compensate for sand left behind on the stoss side) if there were a stream of sand blowing over all the bedforms (traveling faster than the bedforms), from which each bedform might obtain what it needs to maintain its volume and shape. Wind-ripples might operate this way.

Fig 6. Gamma distribution (8, 0.125)
Studies of small (tens of centimeters) subaqueous bedforms in flumes and rivers that have slipfaces (and thus are called dunes) indicate that the depths of successive troughs are highly variable, with the depth of troughs described by a probability density function best fit by a gamma distribution (figure 6), where the right-side tail of the distribution corresponds to deeper troughs. This distribution indicates that while most trough depths cluster around a certain value, a few can be quite deeper. (Of course trough depth variation is only free when the dunes are travelling on an erodible homogeneous substrate, eg., deep sand -- and, in the case of aeolian dunes, dry sand.)
In this model, the gamma distribution's right tail was truncated at about five times its modal value. It's a 'heavy tail', in that it decays more slowly than an exponential curve.
Figure 7 shows what happens if bedform of fixed geometry and unequal trough depths are translated up (by aggradation) and right (by migration). The model is initialized with a train of dunes with trough depths chosen randomly from the gamma probability distribution illustrated in figure 6. The trough tracks are still parallel (because the trough depths aren't time-varying in this configuration) but strata are no longer equally thick, reflecting the unequal differences between successive troughs. Notice that some trough paths are terminated by a subsequent trough that happens to be deeper (eg., the second trough from the left terminates the trough-lines of the two troughs ahead of it, and nearly three ahead).

Fig 7. Climbing translatent strata, produced by (unmaintainable) bedforms of fixed geometry and unequal troughs
Bedform trains in nature with varying trough depths likely do not maintain a fixed geometry. Most sand passing over the brink of a dune with a slipface is captured by the slipface; such a dune 'travels' by the transfer of sand from its upwind stoss slope to its slipface (like the tread on a bulldozer or snowmobile). If the trough lee of a dune is deep, that dune's slipface will be longer and the dune will travel slower than if the leeward trough were shallower. If each dune receives sand only from its stoss slope (likely because the slipfaces of upwind dunes capture sand), then dunefields with troughs of varying depth will have dunes travelling various speeds. Dunes will overrun each other, and trough depths will vary with time -- as examined next.
Figure 8 shows the effect of time-varying trough depth. The model accomplishes this in a simplistic way; each trough is initially assigned two depth values from the gamma distribution, and the trough depth is made to make a sinusoidal transition between the two values as it migrates. (Of course this probably isn't natural but is an improvement over the configuration that generated figure 7.) The transition speed and phase is selected randomly. Note that the gamma distribution shape of the trough depth probability function is such that most trough depth pairs will be both near the mean and thus most troughs will pass without much change. Only occasionally will one of the two trough depth values be extreme, and in that case, there will be a large change in depth as the trough travels.
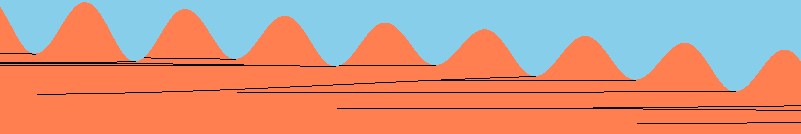
Fig 8. Bedforms with unequal troughs that vary with time
In figure 8 above, most troughs were near the mean and didn't change much as they travel, but one trough made a larger transition during its migration and thus left a much more inclined bounding surface. Here, figure 9, a trough's trajectory is a matter of chance, governed by the shape of the probability distribution function.
Fig 9. Troughs with time-varying depths and trajectories
The aggradation rate for the model runs above (figure 8) was set high so that the trough paths would be well spaced. More reasonable aggradation rates result in paths that tend to overlap and hence erase previous strata, as you can see by playing the video clip to the right.
The thicknesses and shapes of the resulting strata in this simple model is controlled the trough depth probability density function, the aggradation and migration rates, and the way in which troughs change depth with time (their 'trajectory'). The rate of change of trajectories strongly affects the shape of the strata.
When there is variation in trough scour depths, strata can be created even in conditions of no aggradation.
Figure 10 below shows snapshots of the model's strata when there is no aggradation, after the passage of 1000 dunes (top figure), then 2000, 3000, and 4000.
Notice that the strata patterns, in each case, seem similar, and thus unrelated to the number of dunes that passed. It is difficult or impossible to tell by looking at these strata how many dunes (how much time) has passed between these snapshots.

Fig 10. Snapshots after 1000, 2000, 3000, and 4000 dune passages; no aggradation
At a chosen aggradation rate, it takes about 200 dune passages for the model to accumulate the thickness of the sets in figure 11. However, after about 100 dune passages (about half-way, vertically), the aggradation rate was temporarily set to zero, and then one thousand dunes were passed, and then the aggradation rate returned to the original, accumulating the remaining vertical height. Notice that in figure 11 it is not obvious where the episode of no aggradation occurred, during which 1000 dunes passed.

Fig 11. Midway, the aggradation rate was temporarily set to zero, during which 1000 dunes passed
Even though it is known where the mean bedform elevation was during the passage of the 1000 dunes, the maximum scour depth relative to that level at any particular lateral point after the passage of those 1000 dunes depends on chance, as governed by the gamma probability distribution.
A dune contributes to the stratigraphic record only if its deposits are not eroded by subsequent dune troughs. The chart below (figure 12) shows a stratigraphy with aggradation, where the difference between ages (in terms of dune passages) on each side of bounding surfaces was tracked. The segments in red have a difference of 11 (chosen arbitrarily) dune-passes; the dune that scoured those red bounding surface segments was 11 dunes more recent than the dune that left the deposit on the other side of that segment (so 11 dunes are 'missing' there).

Fig 12. Time-varying trough depth, with aggradation. Bounding surface segments dividing deposits with an age difference of eleven dune-passages are highlighted in red.
Figure 13 below shows which dune passes left deposits in the final stratigraphy, where the x-axis is the same as in figure 12, and the y-axis is time (dune passes), increasing upward (298 dunes). Black areas indicate time in which a dune's deposit was subsequently eroded and did not appear in the final record. The record comes from deposits during about 20% of the travel; most of the time (~80%), a dune's deposit is later eroded. Of course the percentages, like the stratigraphy, depend upon model parameters and the model.

Fig 13. Analysis of dune contributions to the stratigraphy in figure 12. Vertical axis is time (dune passage, one/pixel), increasing upward. Orange pixels indicate contributions to the final stratigraphy. Most of the time (~80%), a dune's deposit is later eroded (black pixels).
In the model, where the depth of scour troughs between dunes varies as the dunes travel, we find the following:
Jim Elder
2009 Mar
Jerolmack DJ, Mohrig D, 2005. Frozen dynamics of migrating bed forms. Geology 33: 57-60.
Leclair SF, 2002. Preservation of cross-strata due to migration of subaqueous dunes: An experimental investigation. Sedimentology 49: 1157-1180.